Prime proprietà della trasformata di Fourier

Simmetria coniugata

Nel caso in cui x$\left(\vphantom{ t}\right.$t$\left.\vphantom{ t}\right)$ sia reale, risulta^3.1

X$$\left(\vphantom{ f}\right.$$f$$\left.\vphantom{ f}\right)$$ = X^*$$\left(\vphantom{ -f}\right.$$ - f$$\left.\vphantom{ -f}\right)$$

e quindi la parte reale di X$\left(\vphantom{ f}\right.$f$\left.\vphantom{ f}\right)$ è pari, e quella immaginaria dispari, ossia modulo $\left\vert\vphantom{ X\left( f\right) }\right.$X$\left(\vphantom{ f}\right.$f$\left.\vphantom{ f}\right)$ $\left.\vphantom{ X\left( f\right) }\right\vert$ pari e fase arg$\left\{\vphantom{ X\left( f\right) }\right.$X$\left(\vphantom{ f}\right.$f$\left.\vphantom{ f}\right)$ $\left.\vphantom{ X\left( f\right) }\right\}$ dispari.

Dualità

Trasformata ed antitrasformata differiscono solo per il segno. Ciò comporta che se sostituiamo alla variabile f del risultato X$\left(\vphantom{ f}\right.$f$\left.\vphantom{ f}\right)$ di una $\mathcal {F}$-trasformata, la variabile t, si ottiene una funzione del tempo X$\left(\vphantom{ t}\right.$t$\left.\vphantom{ t}\right)$ che, se nuovamente trasformata, fornisce ... il segnale originario x$\left(\vphantom{ t}\right.$t$\left.\vphantom{ t}\right)$, calcolato nella variabile f, cambiata di segno: x$\left(\vphantom{ -f}\right.$ - f$\left.\vphantom{ -f}\right)$. Il concetto esposto, verificabile analiticamente con facilità, si riassume come

x$\left(\vphantom{ t}\right.$t$\left.\vphantom{ t}\right)$$\;\stackrel{{\mathcal{F}\left\{ \right\} }}{\rightarrow }\;$X$\left(\vphantom{ f}\right.$f$\left.\vphantom{ f}\right)$    $\;\stackrel{t=f}{\rightarrow }\;$    X$\left(\vphantom{ t}\right.$t$\left.\vphantom{ t}\right)$$\;\stackrel{{\mathcal{F}\left\{ \right\} }}{\rightarrow }\;$x$\left(\vphantom{ -f}\right.$ - f$\left.\vphantom{ -f}\right)$

X$\left(\vphantom{ f}\right.$f$\left.\vphantom{ f}\right)$$\;\stackrel{{\mathcal{F}^{-1}\left\{ \right\} }}{\rightarrow }\;$x$\left(\vphantom{ t}\right.$t$\left.\vphantom{ t}\right)$    $\;\stackrel{f=t}{\rightarrow }\;$    x$\left(\vphantom{ f}\right.$f$\left.\vphantom{ f}\right)$$\;\stackrel{{\mathcal{F}^{-1}\left\{ \right\} }}{\rightarrow }\;$X$\left(\vphantom{ -t}\right.$ - t$\left.\vphantom{ -t}\right)$

e consente l'uso di risultati ottenuti ``in un senso'' (ad es. da tempo a frequenza) per derivare senza calcoli i risultati nell'altro (o viceversa).

Esempio: Trasformata di un sinc(t)

Supponiamo di voler $\mathcal {F}$-trasformare il segnale x$\left(\vphantom{ t}\right.$t$\left.\vphantom{ t}\right)$ = B${\frac{\sin \left( \pi tB\right) }{\pi tB}}$ = Bsinc$\left(\vphantom{ tB}\right.$tB$\left.\vphantom{ tB}\right)$: l'applicazione cieca dell'integrale che definisce la trasformata di Fourier appare un'impresa ardua...

 

0.500000

 

 Allora, ricordando che $\mathcal {F}$$\left\{\vphantom{ rect_{\tau }\left( t\right) }\right.$rect_$\tau$$\left(\vphantom{ t}\right.$t$\left.\vphantom{ t}\right)$ $\left.\vphantom{ rect_{\tau }\left( t\right) }\right\}$ = $\tau$sinc$\left(\vphantom{ f\tau }\right.$f$\tau$ $\left.\vphantom{ f\tau }\right)$, scriviamo direttamente che

$$\mathcal {F}$$$$\left\{\vphantom{ B\hbox {sinc}\left( tB\right) }\right.$$Bsinc$$\left(\vphantom{ tB}\right.$$tB$$\left.\vphantom{ tB}\right)$$ $$\left.\vphantom{ B\hbox {sinc}\left( tB\right) }\right\}$$ = rect_B$$\left(\vphantom{ f}\right.$$f$$\left.\vphantom{ f}\right)$$

Pertanto la trasformata di un ${\frac{\sin x}{x}}$ nel tempo, è un rettangolo in frequenza.

Linearità

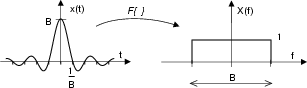
Discende molto semplicemente dalla proprietà distributiva dell'integrale che definisce la trasformata. Pertanto:

se    z$$\left(\vphantom{ t}\right.$$t$$\left.\vphantom{ t}\right)$$ = ax$$\left(\vphantom{ t}\right.$$t$$\left.\vphantom{ t}\right)$$ + by$$\left(\vphantom{ t}\right.$$t$$\left.\vphantom{ t}\right)$$    allora    Z$$\left(\vphantom{ f}\right.$$f$$\left.\vphantom{ f}\right)$$ = aX$$\left(\vphantom{ f}\right.$$f$$\left.\vphantom{ f}\right)$$ + bY$$\left(\vphantom{ f}\right.$$f$$\left.\vphantom{ f}\right)$$

Valore medio e valore iniziale

Subito verificabile una volta notato che la $\mathcal {F}$-trasformata, calcolata per f = 0, si riduce all'integrale di x$\left(\vphantom{ t}\right.$t$\left.\vphantom{ t}\right)$, e quindi al suo valor medio. Pertanto:

m_x = $$\int^{\infty }_{-\infty }$$x$$\left(\vphantom{ t}\right.$$t$$\left.\vphantom{ t}\right)$$dt = X$$\left(\vphantom{ f=0}\right.$$f = 0$$\left.\vphantom{ f=0}\right)$$    e, per dualita':    x₀ = x$$\left(\vphantom{ t=0}\right.$$t = 0$$\left.\vphantom{ t=0}\right)$$ = $$\int^{\infty }_{-\infty }$$X$$\left(\vphantom{ f}\right.$$f$$\left.\vphantom{ f}\right)$$df

dove l'ultima relazione esprime la proprietà del valore iniziale.

Come esempio di applicazione, troviamo subito che

$$\int^{\infty }_{-\infty }$$sinc$$\left(\vphantom{ tB}\right.$$tB$$\left.\vphantom{ tB}\right)$$dt = $${\frac{1}{B}}$$rect_B$$\left(\vphantom{ f=0}\right.$$f = 0$$\left.\vphantom{ f=0}\right)$$ = $${\frac{1}{B}}$$

Traslazione nel tempo

Si tratta di una proprietà molto semplice, e che ricorre frequentemente nei calcoli sui segnali. Manifesta la relazione esistente tra la trasformata dei segnali e quella degli stessi translati, e si esprime con il predicato:

$$\left(\vphantom{ t}\right. \left.\vphantom{ t}\right) \left(\vphantom{ t-T}\right. \left.\vphantom{ t-T}\right)$$ se

$$\left(\vphantom{ f}\right. \left.\vphantom{ f}\right) \left(\vphantom{ f}\right. \left.\vphantom{ f}\right) \pi$$ allora

la cui dimostrazione è fornita sotto^3.2.

Esempio

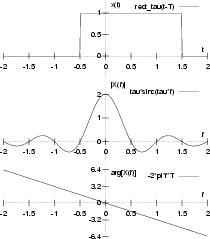
La figura in fondo alla pagina precedente esemplifica il risultato ottenuto per x$\left(\vphantom{ t}\right.$t$\left.\vphantom{ t}\right)$ = rect_$\tau$$\left(\vphantom{ t-T}\right.$t - T$\left.\vphantom{ t-T}\right)$, mostrando come nello spettro di x$\left(\vphantom{ t}\right.$t$\left.\vphantom{ t}\right)$, di modulo $\left\vert\vphantom{ X\left( f\right) }\right.$X$\left(\vphantom{ f}\right.$f$\left.\vphantom{ f}\right)$ $\left.\vphantom{ X\left( f\right) }\right\vert$ = $\mathcal {F}$$\left\{\vphantom{ rect_{\tau }}\right.$rect_$\tau$$\left.\vphantom{ rect_{\tau }}\right\}$ = $\tau$sinc$\left(\vphantom{ f\tau }\right.$f$\tau$ $\left.\vphantom{ f\tau }\right)$, si aggiunga un contributo di fase lineare $\varphi$$\left(\vphantom{ f}\right.$f$\left.\vphantom{ f}\right)$ = - 2$\pi$fT. Nel caso in figura, si è posto $\tau$ = 2 e T = .5, ottenendo in definitiva Z$\left(\vphantom{ f}\right.$f$\left.\vphantom{ f}\right)$ = X$\left(\vphantom{ f}\right.$f$\left.\vphantom{ f}\right)$e^-j2$\pi$fT = 2sinc$\left(\vphantom{ 2f}\right.$2f$\left.\vphantom{ 2f}\right)$e^-j2$\pi$f.

Poniamo ora l'attenzione sul fatto che l'espressione x$\left(\vphantom{ t-T}\right.$t - T$\left.\vphantom{ t-T}\right)$ indica un ritardo del segnale x$\left(\vphantom{ t}\right.$t$\left.\vphantom{ t}\right)$ di una quantità pari a T.

La circostanza che questo ritardo si traduca in un andamento lineare della fase^3.3 di X$\left(\vphantom{ f}\right.$f$\left.\vphantom{ f}\right)$ ha una conseguenza notevole anche nell'altra direzione, ossia:

Una alterazione della fase di X$\left(\vphantom{ f}\right.$f$\left.\vphantom{ f}\right)$ deve essere lineare in f, se si desidera che il segnale mantenga inalterata la sua forma d'onda.

Esempio

Consideriamo un segnale periodico x$\left(\vphantom{ t}\right.$t$\left.\vphantom{ t}\right)$ costituito da due sole armoniche: x$\left(\vphantom{ t}\right.$t$\left.\vphantom{ t}\right)$ = asin$\left(\vphantom{ \omega t}\right.$$\omega$t$\left.\vphantom{ \omega t}\right)$ + bsin$\left(\vphantom{ 2\omega t}\right.$2$\omega$t$\left.\vphantom{ 2\omega t}\right)$ (avendo posto 2$\pi$F = $\omega$), assieme alla sua versione ritardata x$\left(\vphantom{ t-T}\right.$t - T$\left.\vphantom{ t-T}\right)$ = asin$\left(\vphantom{ \omega \left( t-T\right) }\right.$$\omega$$\left(\vphantom{ t-T}\right.$t - T$\left.\vphantom{ t-T}\right)$ $\left.\vphantom{ \omega \left( t-T\right) }\right)$ + bsin$\left(\vphantom{ 2\omega \left( t-T\right) }\right.$2$\omega$$\left(\vphantom{ t-T}\right.$t - T$\left.\vphantom{ t-T}\right)$ $\left.\vphantom{ 2\omega \left( t-T\right) }\right)$ = asin$\left(\vphantom{ \omega t-\omega T}\right.$$\omega$t - $\omega$T$\left.\vphantom{ \omega t-\omega T}\right)$ + bsin$\left(\vphantom{ 2\omega t-2\omega T}\right.$2$\omega$t - 2$\omega$T$\left.\vphantom{ 2\omega t-2\omega T}\right)$. Ponendo $\omega$T = $\theta$, otteniamo x$\left(\vphantom{ t-T}\right.$t - T$\left.\vphantom{ t-T}\right)$ = asin$\left(\vphantom{ \omega t-\theta }\right.$$\omega$t - $\theta$ $\left.\vphantom{ \omega t-\theta }\right)$ + bsin$\left(\vphantom{ 2\omega t-2\theta }\right.$2$\omega$t - 2$\theta$ $\left.\vphantom{ 2\omega t-2\theta }\right)$, e verifichiamo che la seconda armonica subisce un ritardo di fase esattamente doppio.

Figura: Confronto tra diversi spettri di fase

   

In fig 3.2 si è posto a = 1; b = .5; $\theta$ = ${\frac{\pi }{4}}$ e F = .2, ed è mostrato sia il segnale somma originario, sia quello ottenuto considerando un contributo di fase lineare per le due armoniche. Verifichiamo che nel secondo caso, la forma d'onda è la stessa ottenibile per T = 0, in quanto le armoniche sono traslate del medesimo intervallo temporale. A destra invece, la fase della seconda armonica viene annullata, ottenendo dalla somma un segnale asin$\left(\vphantom{ 2\pi Ft-\theta }\right.$2$\pi$Ft - $\theta$ $\left.\vphantom{ 2\pi Ft-\theta }\right)$ + bsin$\left(\vphantom{ 2\pi 2Ft}\right.$2$\pi$2Ft$\left.\vphantom{ 2\pi 2Ft}\right)$. Come è evidente, in questo caso il risultato assume una forma completamente diversa^3.4.

Traslazione in frequenza (Modulazione)

E' la proprietà duale della precedente, e stabilisce che

se    Z$$\left(\vphantom{ f}\right.$$f$$\left.\vphantom{ f}\right)$$ = X$$\left(\vphantom{ f-f_{0}}\right.$$f - f₀$$\left.\vphantom{ f-f_{0}}\right)$$    allora    z$$\left(\vphantom{ t}\right.$$t$$\left.\vphantom{ t}\right)$$ = x$$\left(\vphantom{ t}\right.$$t$$\left.\vphantom{ t}\right)$$e^j2$\pi$f₀t

la cui dimostrazione è del tutto analoga a quanto già visto. Da un punto di vista mnemonico, distinguiamo la traslazione temporale da quella in frequenza per il fatto che, nel primo caso, i segni della traslazione e dell'esponenziale complesso sono uguali, e nel secondo, opposti.

Da un punto di vista pratico, può sorgere qualche perplessità per la comparsa di un segnale complesso nel tempo. Mostriamo però che anti-trasformando uno spettro ottenuto dalla somma di due traslazioni opposte, si ottiene un segnale reale:

$$\mathcal {F}$$^-1$$\left\{\vphantom{ X\left( f-f_{0}\right) +X\left( f+f_{0}\right) }\right.$$X$$\left(\vphantom{ f-f_{0}}\right.$$f - f₀$$\left.\vphantom{ f-f_{0}}\right)$$ + X$$\left(\vphantom{ f+f_{0}}\right.$$f + f₀$$\left.\vphantom{ f+f_{0}}\right)$$ $$\left.\vphantom{ X\left( f-f_{0}\right) +X\left( f+f_{0}\right) }\right\}$$ = x$$\left(\vphantom{ t}\right.$$t$$\left.\vphantom{ t}\right)$$e^j2$\pi$f₀t + x$$\left(\vphantom{ t}\right.$$t$$\left.\vphantom{ t}\right)$$e^{-j2$\pi$f₀t} = 2x$$\left(\vphantom{ t}\right.$$t$$\left.\vphantom{ t}\right)$$cos 2$$\pi$$f₀t

Pertanto, lo sdoppiamento e la traslazione di X$\left(\vphantom{ f}\right.$f$\left.\vphantom{ f}\right)$ in $\pm$f₀ sono equivalenti ad un segnale cosinusoidale di frequenza f₀, la cui ampiezza è modulata dal segnale x$\left(\vphantom{ t}\right.$t$\left.\vphantom{ t}\right)$ = $\mathcal {F}$^-1$\left\{\vphantom{ X\left( f\right) }\right.$X$\left(\vphantom{ f}\right.$f$\left.\vphantom{ f}\right)$ $\left.\vphantom{ X\left( f\right) }\right\}$. E' proprio per questo motivo, che la proprietà è detta anche di modulazione (vedi anche a pag. ).

Coniugato

Deriva direttamente dalla definizione di $\mathcal {F}$-trasformata:

$$\mathcal {F}$$$$\left\{\vphantom{ x^{*}\left( t\right) }\right.$$x^*$$\left(\vphantom{ t}\right.$$t$$\left.\vphantom{ t}\right)$$ $$\left.\vphantom{ x^{*}\left( t\right) }\right\}$$ = X^*$$\left(\vphantom{ -f}\right.$$ - f$$\left.\vphantom{ -f}\right)$$;        $$\mathcal {F}$$^-1$$\left\{\vphantom{ X^{*}\left( f\right) }\right.$$X^*$$\left(\vphantom{ f}\right.$$f$$\left.\vphantom{ f}\right)$$ $$\left.\vphantom{ X^{*}\left( f\right) }\right\}$$ = x^*$$\left(\vphantom{ -t}\right.$$ - t$$\left.\vphantom{ -t}\right)$$

Nel caso di segnali reali, ritroviamo la proprietà di simmetria coniugata.

Cambiamento di scala

Quantifica gli effetti sullo spettro di una variazione nella velocità di scorrimento del tempo (e viceversa). Possiamo pensare come, riavvolgendo velocemente un nastro magnetico, si ascolta un segnale di durata più breve, e dal timbro più acuto. Questo fenomeno viene espresso analiticamente come:

$$\mathcal {F}$$$$\left\{\vphantom{ x\left( at\right) }\right.$$x$$\left(\vphantom{ at}\right.$$at$$\left.\vphantom{ at}\right)$$ $$\left.\vphantom{ x\left( at\right) }\right\}$$ = $${\frac{1}{a}}$$X$$\left(\vphantom{ \frac{f}{a}}\right.$$$${\frac{f}{a}}$$ $$\left.\vphantom{ \frac{f}{a}}\right)$$

in cui scegliendo a > 1 si ottiene accelerazione temporale ed allargamento dello spettro (ed il contrario con a < 1). La dimostrazione è riportata alla nota^3.5.

Prima di continuare ad esporre altre proprietà notevoli della trasformata di Fourier, occorre definire ed analizzare le proprietà della ``funzione`` impulso matematico, indicata con $\delta$(.).